此条目没有列出任何参考或来源。 (2021年5月25日)
维基百科所有的内容都应该可供查证。请协助补充可靠来源以改善这篇条目。无法查证的内容可能会因为异议提出而移除。 |
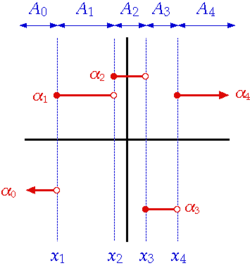
在数学中,如果实数域上的某个函数可以用半开区间上的指示函数的有限次线性组合来表示,那么这个函数就是阶跃函数。换一种不太正式的说法就是,阶跃函数是有限段分段常数函数的组合。
假设已知:



 (对于
(对于 )
)
(尽管这个例子中的区间下边界包含在内,而上边界不包含在内,但是这并不是定义所要求的。只要区间 An 互不相交,并且它们的组合是实数就可以了。)
定义: 函数  是 阶跃函数的条件是当且仅当它可以表示为
是 阶跃函数的条件是当且仅当它可以表示为
对于所有 有
有
 其中
其中  是
是  的指示函数:
的指示函数:

注意: 对于所有的  及
及  满足:
满足: