英国的海岸线有多长？统计自相似和分数维度

《英国的海岸线有多长？统计自相似和分数维度》（英语：How Long Is the Coast of Britain? Statistical Self-Similarity and Fractional Dimension），是由法国、美国数学家本华·曼德博（Benoît B. Mandelbrot）撰写的论文，最初在1967年于《科学》发表^[1]。在这篇论文内曼德博讨论了维度于1和2之间的自相似曲线。虽然曼德博没有使用分形（fractal）这个词汇^[2]，惟这些曲线均为分形。

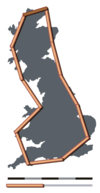
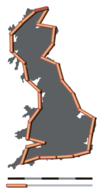
论文的首部分，曼德博讨论了英国数学家路易斯·弗莱·理查德森（Lewis Fry Richardson）对海岸线与其他自然地理边界的测量出来的长度如何依赖测量尺度的研究。理查森观察到，不同国家边界测量出来的长度${\displaystyle L(G)}$是测量尺度${\displaystyle G}$的一个函数。他从不同的好几个例子里搜集资料，然后猜想${\displaystyle L(G)}${\displaystyle L(G)} 可以透过以下形式的一个函数来估计：

${\displaystyle L(G)=MG^{1-D}}$

曼德博将此结果诠释成显示海岸线和其他地理边界可有统计自相似的性质，而指数${\displaystyle D}$则计算边界的豪斯道夫维度（Hausdorff-Besicovitch Dimension）。透过这个看法，理查森的研究的例子的有着从南非海岸线的1.02到英国西岸的1.25的维度。

在论文的第二部分，曼德博描述了不同的关于科赫雪花的曲线，它们都是标准的自相似图形。曼德博显示计算它们的豪斯道夫维度的方法，它们的维度都是1和2之间。他亦提及填满空间、维度为2的皮亚诺曲线，但并未给出其构造。

这篇论文很重要，因为它既显示了曼德博早期对分形的思想^[3]，同时又是数学物件和自然形式的联结的例子——曼德博以后很多工作的主题。